Since I'm tutoring Topology this semester, I thought it would be a good idea to make a post on something introductory of Topology. So, I remembered the following exercise of the very beginning of the course:
Exercise: Let $\tau$ and $\tau'$ be topologies on a set $X$. Then, the identity map $(X, \tau) \hookrightarrow (X,\tau ')$ is continuous iff $\tau' \subset \tau$.
The proof is trivial. As a corollary, one gets:
Corollary: $\tau = \tau'$ iff the identity is a homeomorphism.
All of the above are easy results that seem to be only mild exercises. We now show that they furnish a way to understand how rigid a compact hausdorff topology is.
For that, consider the following observations which follow from the definitions.
OBS1: If $(X,\tau)$ is hausdorff and $\tau \subset \tau'$, then $(X,\tau')$ is hausdorff.
OBS2: If $(X,\tau')$ is compact and $\tau \subset \tau'$, then $(X,\tau)$ is compact.
Now we present the only non-trivial lemma of this post (although it is quite straight-forward):
Lemma: Every continuous bijective function from a compact space to a hausdorff space is a homeomorphism.
Proof: It suffices to prove that image of closed sets is closed. Take a closed set on the domain. Since it is closed on a compact space, it is compact. Its image is therefore compact. But a compact set in a hausdorff space is closed. $\blacksquare$
Now we come to the crux of the post:
Proposition: Let $(X,\tau)$ be a compact hausdorff space. If $\tau' \subsetneq \tau$, then $(X,\tau')$ is not hausdorff. If $\tau' \supsetneq \tau$, then $(X,\tau')$ is not compact.
In other words, if you take off open sets of $\tau$ you lose hausdorffness and if you put new open sets in $\tau$ you lose compactness.
Proof: The reader may want to prove this on his own. If not, go ahead.
Let's prove the first case. Suppose $(X,\tau')$ is hausdorff. By OBS2, we have that $(X,\tau')$ is compact. Hence, by the exercise together with the lemma, we have that the identity is a homeomorphism. By the corollary of the exercise, this implies $\tau' =\tau$, a contradiction. Similarly, we have the second case. $\blacksquare$
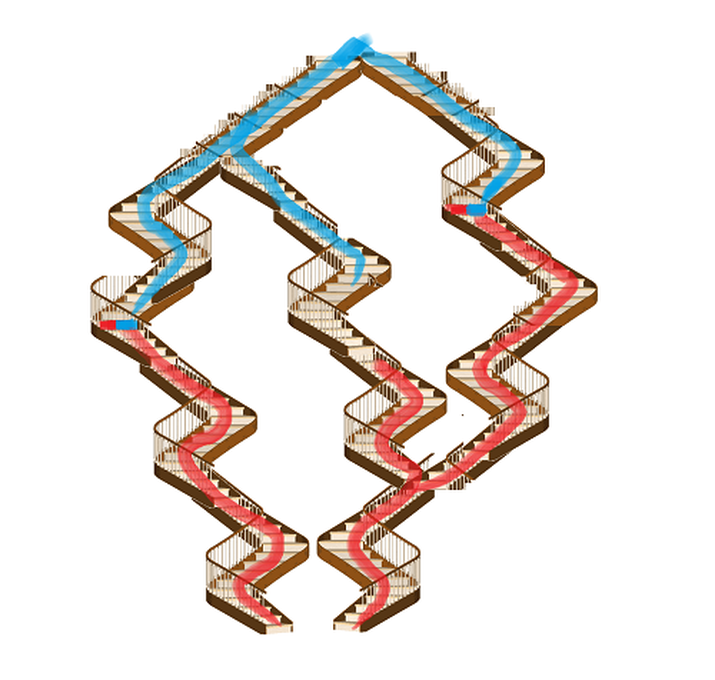
This can be illustrated with as follows:
Imagine we have a flight of stairs. Each step of the stair is a topology on a given set $X$. The bottom is the trivial topology, the top is the discrete topology. A red step means a topology which makes $X$ compact, and a blue step is a topology which makes $X$ hausdorff. What the theorem above says is that in a given "line" of stairs, there can be at most one step which is both red and blue.
 RSS Feed
RSS Feed
